For everybody getting here from google, like me: the log$\log$ might have been replaced in the loss function, but I think it is still there when taking the gradient of both functions (correct me, if I am wrong):
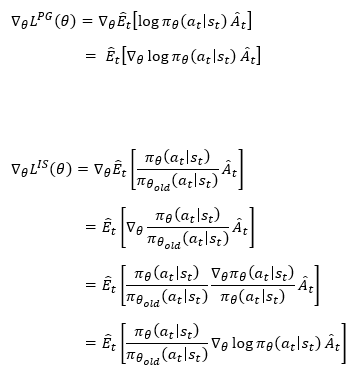
$$\begin{aligned} \nabla_{\theta} L^{P G}(\theta) &=\nabla_{\theta} \hat{E}_{t}\left[\log \pi_{\theta}\left(a_{t} \mid s_{t}\right) \hat{A}_{t}\right] \\ &=\hat{E}_{t}\left[\nabla_{\theta} \log \pi_{\theta}\left(a_{t} \mid s_{t}\right) \hat{A}_{t}\right] \end{aligned}$$
and
$$ \begin{aligned} \nabla_{\theta} L^{I S}(\theta)=& \nabla_{\theta} \hat{E}_{t} \left[\frac{\pi_{\theta}\left(a_{t} \mid s_{t}\right)}{\pi_{\theta_{\text {old}}}\left(a_{t} \mid s_{t}\right)} \hat{A}_{t}\right] \\ &=\hat{E}_{t} \left[\nabla_{\theta} \frac{\pi_{\theta}\left(a_{t} \mid s_{t}\right)}{\pi_{\theta_{\text {old}}}\left(a_{t} \mid s_{t}\right)} \hat{A}_{t}\right] \\ &=\hat{E}_{t} \left[\frac{\pi_{\theta}\left(a_{t} \mid s_{t}\right)}{\pi_{\theta_{\text {old}}}\left(a_{t} \mid s_{t}\right)} \frac{\nabla_{\theta} \pi_{\theta}\left(a_{t} \mid s_{t}\right)}{\pi_{\theta}\left(a_{t} \mid s_{t}\right)} \hat{A}_{t}\right] \\ &=\hat{E}_{t}\left[\frac{\pi_{\theta}\left(a_{t} \mid s_{t}\right)}{\pi_{\theta_{\text {old}}}\left(a_{t} \mid s_{t}\right)} \nabla_{\theta} \log \pi_{\theta}\left(a_{t} \mid s_{t}\right) \hat{A}_{t}\right] \end{aligned} $$
So, the log$\pi_{\theta}\left(a_{t} \mid s_{t}\right) $ in the PG function was replaced with r(theta)$\frac{\pi_{\theta}\left(a_{t} \mid s_{t}\right)}{\pi_{\theta_{\text {old}}}\left(a_{t} \mid s_{t}\right)} $ whose derivate is the same as the loglog of the PG function (appartapart from the prportionalityproportionality factor r(theta)).
