How can we derive the final result? I can understand the first line, but don't know how the absolute term in the summation is replaced with $2\epsilon t$.

https://www.youtube.com/watch?v=LtAt5M_a0dI&list=PL_iWQOsE6TfXxKgI1GgyV1B_Xa0DxE5eH&index=45

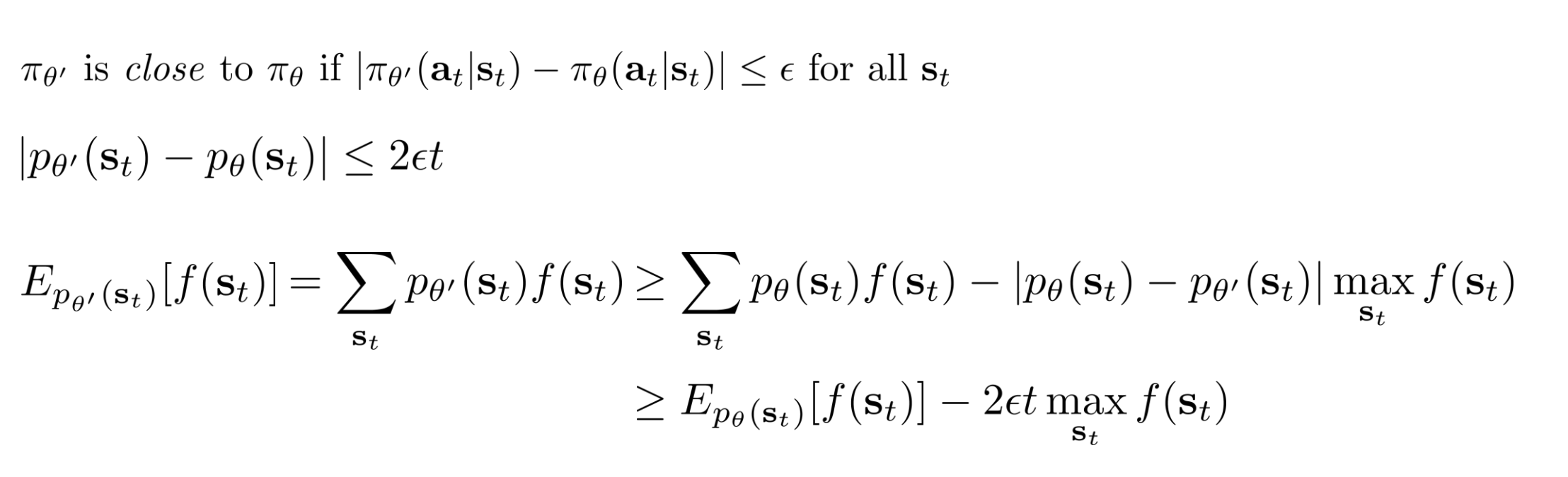
I agree with the $$\sum_{s_t}p_{\theta'}(s_t)f(s_t) \geq \sum_{s_t}\big[p_{\theta}(s_t)f(s_t) - |p_{\theta}(s_t) - p_{\theta'}(s_t)|max_{s_t}f(s_t)\big]$$ $$\because \sum_{s_t}p_{\theta}(s_t)f(s_t) = \sum_{s_t}\big(p_{\theta}(s_t) + p_{\theta'}(s_t) - p_{\theta'}(s_t) \big)f(s_t)$$ $$= \sum_{s_t}p_{\theta'}(s_t)f(s_t) + \big(p_{\theta}(s_t) - p_{\theta'}(s_t) \big)f(s_t)$$ $$ \leq \sum_{s_t}p_{\theta'}(s_t)f(s_t) + \big|p_{\theta}(s_t) - p_{\theta'}(s_t) \big|f(s_t)$$ $$ \leq \sum_{s_t}p_{\theta'}(s_t)f(s_t) + \big|p_{\theta}(s_t) - p_{\theta'}(s_t) \big|max_{s_t}f(s_t)$$

so, $$\sum_{s_t}p_{\theta'}(s_t)f(s_t) \geq \sum_{s_t}\big[p_{\theta}(s_t)f(s_t) - |p_{\theta}(s_t) - p_{\theta'}(s_t)|max_{s_t}f(s_t)\big] \geq \sum_{s_t}\big[p_{\theta}(s_t)f(s_t) - 2\epsilon t max_{s_t}f(s_t)\big] = E_{p_{\theta}(s_t)}[f(s_t)] - \sum_{s_t}2\epsilon t max_{s_t}f(s_t)$$

Here is the question. How does the lecture note remove the summation of the epsilon related term? The professor sasy, since all of them are constant (I understand $max_{s_t}f(s_t)$ is also a constant), we can write like that, but $\sum_{t=1}^{10}(t - 1) = 45 = \sum_{t=1}^{10}t - \sum_{t=1}^{10}1 = 55 - 10 = 45$. We don't write $\sum_{t=1}^{10}1 = 1$ because 1 is a constant.

The abiguous part is there is no concrete size of $s_t$ in the summation, but at least I think just removing the summation in front of the epsilon term is weird.