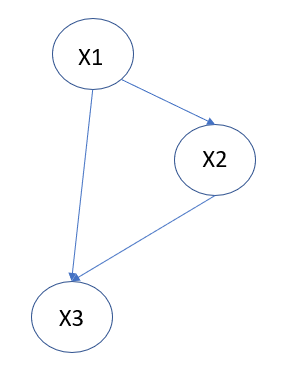
In the diagram below, there are three variables: X3 is a function of (depends on) X1 and X2, X2 also depends on X1. More specifically, X3 = f(X1, x2) and X2 = g(X1). Therefore, X3 = f(X1, g(X1)).
If the probabilistic distribution of X1 is known, is it possible to derive the probabilistic distribution of X3?
Yes you can, provided you know about $f$ and $g$. Expression $X3 = f(X1, g(X1))$can be written as $X3 = h(X1)$ where $h$ takes into account both $f$ and $g$. After this finding the PDF is simple by differentiating the CDF: $$ F_{X3} (x3) = P(X3 \leq x3) = P(h(X1) \leq x3) = P(X1 \leq h^{-1}(x3))$$
$$ \frac {d F_{X3} (x3)}{dx3} = \frac {d P(X1 \leq h^{-1}(x3))}{dx3} = f_{X3}(x3)$$
NOTE: The conventions followed are the same as used in the field of Probablity
(Take care of the function inversion step in non-monotonic cases)
Check these lectures.
No, it is not possible. We could derive the most probable $x_3$ by calculating the maximum likelihood: $x^*_3=\underset{x_3}{\arg\max} p(x_1,x_2|x_3)$. We are unable to calculate this as you only stated that there is a correlation, but we don't know how it looks like.
