According to the provided article,
$$
\begin{equation} \tag{1}
P(D| {\mathcal{E}};\ \theta )
\end{equation}
$$
is a probability of disease $D$ given findings $\mathcal{E}$, and a model $\theta$ that is used to estimate this probability.
$D$ represents a disease or diseases, and findings $\mathcal{E}$ can
include symptoms, tests outcomes and relevant medical history.
The Sheffer stroke symbol (vertical bar) | in the conditional probability notation is read "given that", whereas the semicolon symbol ; tells that we use a model (or parameters for the model) to calculate this probability. For instance, we can define this probability as $P(D| {\mathcal{E}};\ \theta ) = M_{\theta}(\mathcal{E})$, where $M$ can be a nueral network with parameters $\theta$ that takes $\mathcal{E}$ as input and returns the probability of $D$.
As we read the article further, we see that Equation 1 is nothing more than the posterior probability from Bayes' theorem:
$$
\begin{equation} \tag{2}
P(D| {\mathcal{E}};\ \theta )=\frac{P({\mathcal{E}}| D;\ \theta )P(D;\ \theta )}{P({\mathcal{E}};\ \theta )}.
\end{equation}
$$
where $P({\mathcal{E}}| D;\ \theta )$ is a likelihood of findings $\mathcal{E}$ given that we have the disease $D$, $P(D;\ \theta )$ is the prior probability of the disease $D$, and $P({\mathcal{E}};\ \theta )$ is a likelihood of findings $\mathcal{E}$.
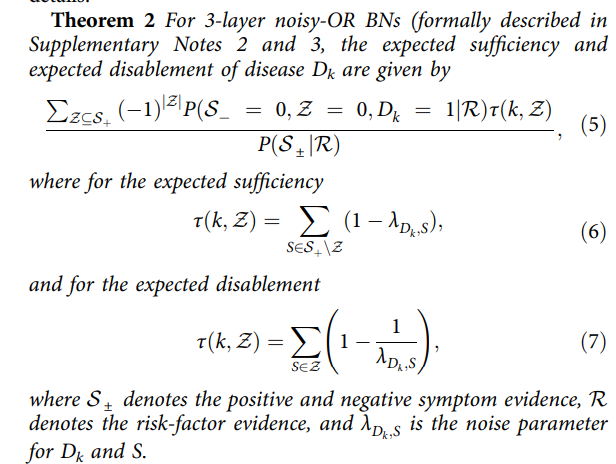
As the article suggests, Theorem 2 is related to the Noisy-OR model, which itself is a large area of research. I encourage you to read the references provided in the article to learn more about approaches used by authors. If you have further questions regarding this theorem, I suggest you to open another question.